There are a lot of named phenomena in paleontology that refer to specific circumstances, which I think are really interesting but are hard to search for. Often they’re patterns or paradoxes named after the individual thinkers who came up with them, sort of like the set of Internet axioms found on TVTropes*. In this post, I’ve collected a variety of concepts for your convenience, with a short overview of each. If you know of any more that deserve to be on this list, let me know and I’ll append it!
The Boring Billion
The Boring Billion was a period of time from about 1.8 billion to 0.8 billion years ago in which “nothing happened.” Eukaryotes (complex single-celled organisms) and sexual reproduction had arisen just before this period, and prokaryotic cyanobacteria had appeared and were pumping out oxygen, but for a billion years, not a lot of obvious change was happening. The earth was tectonically calm and nutrient-poor, with the cyanobacteria causing euxinic (low oxygen, high sulfur) ocean conditions that were harsh for other life.
What was actually going on during this period was that all the oxygen produced by the cyanobacteria was being absorbed into the oceans and rocks, with very little making it into the atmosphere. Finally, around 0.85 billion years ago, the oxygen reserves of the planet were filled and gaseous oxygen was able to exist in the atmosphere, allowing many more diverse eukaryotes to evolve, including the first animals. However, many single-celled organisms were adapted to the oxygen-poor environment, and died out in a mass extinction known as the Great Oxidation Event, with the only survivors continuing to eke out a living in extreme environments such as deep-sea trenches and salt flats.
Romer’s Gap
Romer’s Gap is a period of time from about 360 to 345 million years ago, spanning the Early Carboniferous Period, from which there are very few fossils known. It was named after paleontologist Alfred Romer (1894-1973), who first noticed it. For a long time, it was debated whether the gap was caused by poor fossilization conditions during that time period, actual low populations of animals that were recovering from the end-Devonian extinction, or if we just hadn’t looked in the right places yet. Recently, stem-tetrapod remains from Scotland have been found within the gap, indicating the third option is likely, but the second also probably plays a role.
The fossil ecosystems recovered before the gap are full of placoderms, early “fishapods” like the -stegas (Acanthostega, Ichthyostega, etc), and relatively simple terrestrial plants and arthropods, while those after the gap are way more modern, with complex forests full of specialized amphibians, arthropods, and trees. Figuring out what happened in Romer’s Gap has been a slow process that is still ongoing.
Polydactyly in Early Tetrapods
The earliest tetrapods, or four-limbed animals, were amphibian-like creatures that inhabited the shallows of the Devonian Period. And they often had way too many digits on each hand and foot.
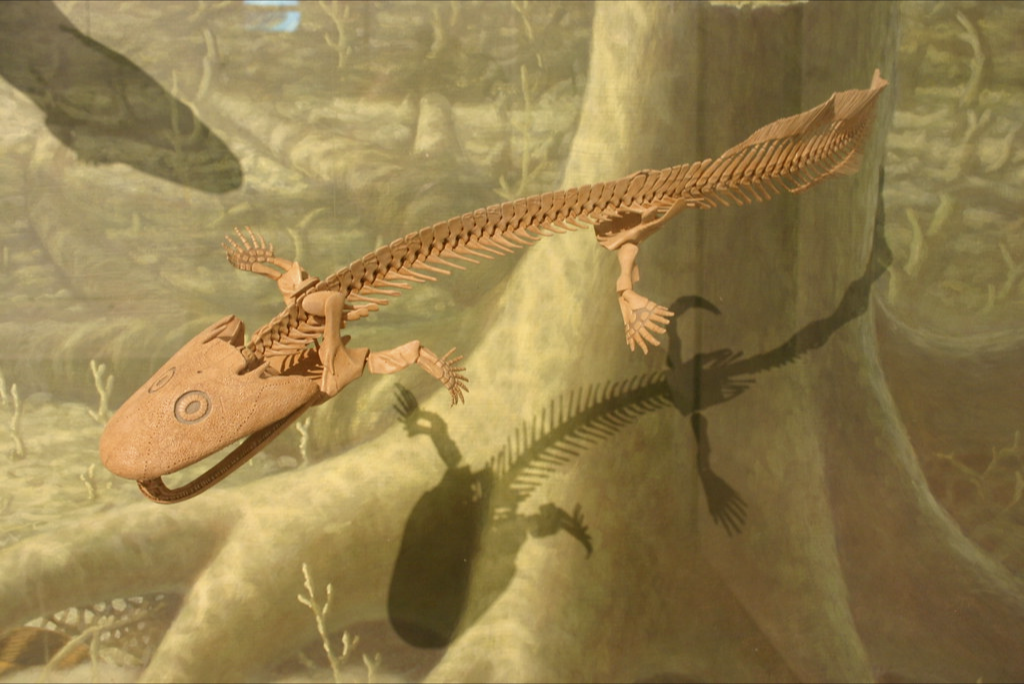
Acanthostega takes the cake with eight digits per foot, but Ichtyostega had a respectable seven and Tulerpeton six. In life, some of these would probably have been fused, giving the outward appearance of fewer digits than in actuality.
But why? It has something to do with the way creating a limb out of a fin happens at the genomic level. Fish have way too many bones in general, and early tetrapods didn’t really have specific requirements for their feet other than supporting their weight.

Above: the skeleton of an anglerfish, showing the many confusing bones that make up its skull, as well as the many ray bones that make up its pectoral fin.
Modern amphibians only have four fingers on their front limbs and five in the back, while the ancestral condition of amniotes (reptiles and mammals) was five on each limb (although this number has been secondarily reduced in many groups such as horses, cows, and birds). For awhile it was unknown whether amniotes and amphibians both evolved from a five-fingered ancestor, or if four fingers was the amphibian ancestral condition as well; new evidence favors the former. While it does seem like the stem-tetrapods themselves were trending toward fewer digits as they became more terrestrial, the fact that all modern tetrapods have the same ancestral number of fingers indicates that maybe our five digits only happened by chance.
The Arthropod Head Problem
Arthropods (insects, crustaceans, arachnids, trilobites, and other hard-shelled, many-legged creatures) are an ancient and diverse group of animals, whose evolutionary relationships and development are still somewhat mysterious. The arthropod head problem, also known as the “endless dispute,” is an ongoing debate about how different groups of arthropods’ head parts are related to one another. Ancestral arthropods are thought to have been simple, segmented creatures, rather like worms, and over time some of the segments specialized or fused into the body parts we see today. The arthropod head problem involves determining, through studying modern arthropod embryos and genetics, which segments in one group correspond to which in another.
Ontogeny Recapitulates Phylogeny
Ontogeny is the way an individual organism grows and develops; phylogeny is the evolutionary relationship of a species to other species. Ontogeny Recapitulates Phylogeny, or just recapitulation, is an erroneous theory that an organism goes through all the stages of its phylogeny as an embryo: for example, a human embryo has gills and a tail like a fish early on in its development, “recapitulating” the human species’ development from fishlike ancestors.
It’s easy to see why this theory was attractive. There are many examples of embryos exhibiting more “primitive” characteristics during development than they end up being born with. A more recent example is the discovery that quail embryos’ hip bones look like those of non-avian dinosaurs before reconfiguring themselves later in development into the normal bird arrangement, “recapitulating” the bird transition from dinosaurs. However, this theory is more about pattern-matching than causality, and is therefore no longer accepted. While embryos do resemble each other early in development, any resemblance to primitive adult forms is coincidental.
Cope’s Rule
Cope’s Rule states that on average, animals tend to increase in body size over time. Bone Warrior Edward Drinker Cope first noticed this trend in Cenozoic mammals: early horses, such as Eohippus, were just a foot tall, while horses as we know them now are obviously larger than that. Early proboscideans (elephant ancestors) like Eritherium were less than a foot tall. Cope hypothesized that large body size is often advantageous, and is selected for until they reach some maximum size dictated by physical constraints, such as elephants’ ability to shed heat (elephants live dangerously close to fatal overheating, and if they were any bigger, couldn’t exist in the hot climates that they currently do).
This can happen in two ways: the average body size of individuals can actually increase, or the number of large types of animal can increase. The former situation is intuitive, while the second takes a little more explanation. For example, consider ten clams, divided into two species of 5 individuals each. Species A weighs 3 grams apiece and Species B weighs 1 gram apiece, for a total average clam weight of 2 grams. But at a later time, Species A has done somewhat better than Species B, increasing their numbers to 8 individuals while Species B reduces to 2 individuals. Now the average clam weight is 2.6 grams, but no individual clam is actually bigger than any from the starting population. Let’s call this “clade selection,” while individuals increasing in body size (like if the Species A clams each became 4 grams in weight) can be “individual selection,” after Hone et al (2005).
While Cope’s Rule does seem intuitively correct, we haven’t had large enough fossil datasets to know if it’s a real rule or a case of asking the wrong statistical question, and if it’s real, how and why it works. Recently, a study published on non-avian dinosaurs has shown a significant expression of Cope’s Rule but the data are too sparse to know whether clade selection or individual selection or both are at work, while a study on sea creatures has shown Cope’s Rule by clade selection. However, there are counterexamples to Cope’s Rule (as confirmed in the above-linked paper, Mesozoic marine invertebrates stayed the same size while Paleozoic and Cenozoic marine invertebrates tended to increase in size) and in general the story is complex, especially since there are many other traits linked to size that could be directly selected for or against, causing size to be indirectly affected. So I’d say Cope’s Rule would more accurately be called “Cope’s Observation”.
The Square-Cube Law
This is more of a property of math, but with many implications for biology. The square-cube law states that as an object increases in size, its surface area increases slower than its volume, so phenomena that depend on surface area or volume will act differently on a small organism versus a big one. Imagine a cube one meter on each side: it has a surface area of six sides times one square meter per side, or six square meters; its volume is just one meter cubed. This puts its surface-area-to-volume ratio at 6:1. A cube two meters on each side has a surface area of six times four square meters per side, or 24 square meters, and its volume is eight cubic meters, meaning its surface-area-to-volume ratio is 24:8, or 3:1. The larger cube has proportionally more volume than the smaller.
A muscle or bone’s strength depends on its cross-sectional area (which scales in the same way as surface area), while its weight depends on its volume. That means as a muscle or bone’s size increases, its relative strength decreases. This is why insects are so proportionally strong and can lift objects many times their weight, while whales can’t even support their own weight outside of water. It’s also why kaiju and humongous mecha should either not exist, or move so slowly as to make battles rather uninteresting.
Another biological realm in which the square-cube law is important is thermoregulation. A warm-blooded animal’s heat generation depends on its volume, while its heat dissipation depends on its surface area, meaning a large animal will have a hard time shedding enough heat to maintain a constant temperature, while a small animal will have a hard time retaining enough heat. That’s why small animals tend to be fluffy while large animals have a sparser integument. This phenomenon is a bit more complicated than the one pertaining to structural strength, because an animal’s thermal adaptations also depend on if it lives in a cold or warm environment, and its shape (if it’s stockier versus lankier). Mark Witton has a great blog post about the relationship between thermal neutrality and body size, and what implications that has for paleoartists who are deciding how much fluff to give an animal.
Bergmann’s Rule
Bergmann’s Rule states that closely-related animals (and maybe even some plants) tend to be larger-bodied in colder locations. The textbook example is Swedish moose, which even within the same species are significantly larger on average the higher-latitude in the country you go. The obvious reasoning calls upon the square-cube law, since larger endothermic animals stay warm better in cold environments, but some ectotherms, such as crocodylians, ants, and marine crustaceans, follow the rule too, while some endotherms, especially small mammals, which the heat-conservation hypothesis predicts should be the strongest conformers to Bergmann’s Rule, do not. So, while there’s evidence of Bergmann’s Rule being a significant trend, heat conservation may only be part of the cause. Other hypotheses include oxygen requirements for aquatic species (colder water tends to have more oxygen, allowing animals to get bigger), reduced predation and competition in colder places, and the greater ability of larger animals to survive periods of resource scarcity, which are more frequent in colder environments. As of yet, no one cause has been accepted as the basis for this rule.
Allen’s Rule
Another thermoregulation-related rule, Allen’s Rule states that animals living in colder environments tend to be stockier, and animals in warmer places are lankier. This is true for humans, bears, seabirds, and others, but there haven’t yet been any comprehensive studies that examine a large dataset to see if the rule holds in general. However, in addition to the fact that stockier animals retain heat better and thus would have a selective advantage in the cold, preliminary research in mice indicates that being raised in the cold actually changes the animals’ proportions as adults. Their blood flow to extremities and cartilage growth is different than mice raised in warm environments, resulting in shorter tails and ears and better heat conservation in the cold-raised mice. I think this is really interesting–most of these “rules” are macro-evolutionary trends that can only be observed across populations across time, but this one might apply to individuals. I hope a large-scale study is done on this soon.
Peto’s Paradox
The likelihood that you get cancer is proportional to the number of cells you have, since each cell has some small, independent chance of becoming cancerous (and then going unnoticed by the body’s regulatory systems). Cancer risk correlates significantly with height in humans and size in dogs (and probably all animals, but studies on humans and dogs are what’s been published so far): taller humans and larger dogs are at higher risk for developing cancer, since they have more cells and thus more chances for cancer to develop. However, between species this relationship doesn’t hold. A whale has many times more cells than a human, which has many times more cells than a mouse, but whales do not contract cancer any more often than mice. This is complicated by the fact that cells come in many sizes (for example, the smallest animal, a parasitic wasp called Dicopomorpha, is less than 150 microns in length and made of hundreds or thousands of cells, while the well-studied single-celled Paramecium can be over 300 microns long) and grow and divide at different rates between different animal species. However, tumor suppression genes have been located in large animals like elephants and whales, which smaller animals lack, indicating that Peto’s Paradox is a real effect that requires further explanation.
Just For Fun
* A quick list of the abovementioned Internet axioms:
- Hanlon’s Razor: “Never attribute to malice that which can be explained equally well by stupidity.”
- Sturgeon’s Law: ”90 percent of everything is c**p.”
- Bellisario’s Maxim: “Don’t examine this too closely.”
- MST3K Mantra: “It’s just a show, we should really just chill out.”
- Godwin’s Law: ”As the length of the comment section increases, the probability of someone comparing someone to Hitler approaches 1.”
- Moff’s Law: “Never try to dismiss critical analysis by asking, ‘Why can’t you just enjoy it for what it is?’”
- Poe’s Law: “If something is sufficiently extreme, it becomes impossible to tell if it’s real or a parody.”
- Haggard’s Law: “The louder and more frequent one’s objections to homosexuality are, the more likely one is to be a homosexual.”
- Greater Internet F**kwad Theory (GIFT): “Regular person + anonymity + audience = total f**kwad.”
- Rule 34: “If it exists, there’s p**n about it.”

